String Bass Effect and MIDI on a C2

We'll continue here with more pictures of this project. Here is a look at the pedal sustain printed circuit board which shows the 37 individual keyers. You might at first say to yourself, "Self, why is Eric using 37 keyers when there are only 25 pedals?" The reason for that however is that I want the string bass to be available at 32', 16' and 8' pitches. This necessitates using a total of 37 pitches altogether because the 8' range goes an octave higher than the 16' range and needs an additional 12 keyers. If I were using the diode keying method found in the pedals of the X66, I would need to use 50 keyers, however by using these transistorized keyers, I can have some of them do double duty. If you are using 16' pitch and play middle C on the pedals, for example, you will use keyer number 13 because with 16' pitch, bottom C on the pedals is number one and Middle C, an octave higher, uses keyer 13. However, if we are using the string bass at 8' pitch, which is the usual pitch at which a string (sustain) bass effect is used, then bottom C pedal plays keyer 13. So we are having certain keyers do double duty.

Figure 4.Here are the pedal sustain keyers, mounted on a hinged plywood panel, the other side of which holds the MIDI multiplexers and encoder cards. The preamp in this picture is the first one I made which is also an experimental one which I used. You will see one trimpot, and several of the resistors and capacitors are likewise removable so that I can make changes to get the best possible performance. The pedal keyer preamp has several important criteria in its design, among which are that it must filter out low frequency keyer thump, high frequency pop and snap sounds, and also pass sufficient bass.

Figure 5. Diode matrix relay that selects appropriate keyers for 32', 16' or 8' string bass pitch. To produce the first octave of 32' pitch the relay will select both a 16' pitch keyer and also one at 10 2/3' pitch. In the second octave, it starts out with keyer 1 playing from pedal 13.

Furthermore, if I am going to have the string bass available at 32' pitch, which is one octave lower than the normal pedal range, I have to resort to an interesting trick known as resultant pitch. If two tones are sounded simultaneously, and if they have a 3:2 frequency ratio, under certain conditions they will react with each other and form a third pitch with a frequency an octave lower than that of the lower of the two original pitches. This effect is often done in pipe organs to produce a 32' bass effect when lack of sufficient space or funding to include real 32' pipes would otherwise eliminate any possibility of having any 32' pedal tone. By having two smaller organ pipes whose pitches have frequencies that are in a 3:2 ratio sound simultaneously, it is possible to get a usable 32' tone from the instrument. If these pipes can be arranged to be fairly physically close together, their soundwaves will intermodulate and generate a pitch whose frequency, considering the 3:2 ratio of the two sounding pipes, will be 1. That is, if you have some event going along at say three times per second, and another event proceeding at two times per second, there will be one place once every second where the two events will occur simultaneously, thus three events in one second plus two events in one second will give one third event (coincidence) once per second.

Seems like we're getting something for nothing here, because we're producing an additional octave of pitches below the lowest available octave of pitches but we haven't added any extra tonewheels to the C2 to make these low tones. But we must add some extra circuitry to make it possible for one pedal to sound two keyers together which have a 3:2 frequency ratio, so there is some extra stuff after all. To do this, we're using a simple diode matrix keying relay, but although we're not getting a "free lunch," we're getting a cheaper lunch in that the diode matrix is a lot simpler and cheaper than a set of special tonewheels to generate true 32' pitches. When low frequency sinusoidal electrical waves are combined in a circuit, amplified together, and sent through the same audio channel and speaker, they will modulate each other sufficiently to create the missing or nonexistent low tones that we need, and thus we are able to sound 3 pitches, each an octave apart, from each key of a 25 note pedal board using 37 keyers in combination with a simple diode matrix keying relay which allows a single pedal to sound multiple keyers simultaneously.

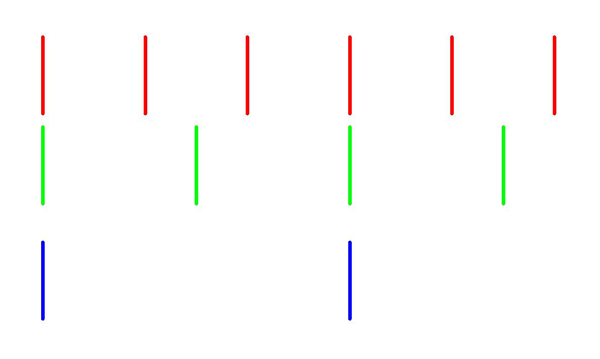
Figure 6. Here's a look at how a series of events (red lines) that occur 6 times in two seconds, and a series of events (green lines) that occur 4 times in two seconds combine to form a series of events [blue lines; coincidences] that occur twice in two seconds. Since 6:4 is actually a 3:2 ratio, this shows graphically how sounds with a 3:2 frequency ratio can produce a third sound with just one cycle for every three cycles of the higher pitch and every two cycles of the lower pitch.

Although this phenomenon happens every time when different frequencies sound together, it is much less noticeable on higher pitches which is why we are not generally aware of it when we play chords and melody, and also why it will work on low frequency pedal bass. The effect is both a physiological result of the way our ears work and also a physical effect, in this case, the way electrical waves of different frequencies can react with each other in a circuit.

In actuality, the frequencies that a Hammond organ produces are not exactly in a 3:2 ratio, because the Hammond is designed to approximate very closely a standard 12 tone equally tempered scale so that it will correspond to all other keyboard musical instruments, however, the resultant effect will work if you are very close to the ideal 3:2 ratio. For example, to produce 32' low CCCC by this method, we will sound the low CCC keyer which is the first and lowest pitch that a Hammond produces and is 32.7 Hz. Along with this, we'll sound the keyer for GGG which is 48.99 Hz. This is a ratio of 2.996:2 As we can see, that is virtually 3:2, because for all intents and purposes we can round off 2.996 to 3.

It's interesting to note that one of Hammond's claims to fame for the traditional Hammond organ is that it would never go out of tune. And essentially this is true. Because the tone wheels are all geared together, unless there is mechanical damage, they will all rotate at precisely the correct speeds relative to one-another. However, the Hammond is not exactly perfectly in tune to begin with. It very closely approximates the equally tempered scale, but because the ratio of one frequency to another in the tempered scale is an irrational number, and because gears must always have an integral number of teeth, the Hammond tuning, which we may correctly call a Hammond temperament, is very close, but not exact. However, the above is strictly academic; Hammond's pitches are extremely close to the ideal tempered scale. The discrepancies amount to such small errors [around two cents in the worst case] that even the most sensitive concert trained musician with really accurate perfect pitch would never be able to hear the errors. The general consensus of opinion is that a person can't perceive any pitch difference if the difference is under 6 cents, a cent in this case being 0.01th of the pitch distance between two frequencies that are a semitone apart on a correctly tuned 12 tone equally tempered musical scale. In reality, there is no such thing as absolutely perfect tuning. The best we can ever expect from anything that is musically tunable is to get a really close approximation. And the tuning of a Hammond organ is a really good and accurate approximation. I would say that even if the world's best piano tuner just spent 4 hours tuning your piano, the typical tonewheel Hammond would still produce pitches that will be closer to the ideal. After all, if 6 cents is the smallest pitch difference that is detectable by a human being, you can't expect a human tuner to be any closer than that; and the greatest tuning accuracy error of a traditional Hammond is only two cents.

The pitch accuracy of the Hammond as a whole is governed by the frequency of the AC that supplies it. Utility supplied power today is very accurately controlled, although it slightly varies throughout the day. However, again we are looking at a theoretical or academic situation. For all general and practical purposes, we may consider that the frequency of utility supplied power is a constant. It is certainly constant enough to govern the tuning of a musical instrument. As a point of interest, A55, A 110, A220, A440, A 880, A 1760 and A 3520 are, if the incoming power is 60Hz, all exactly true, with no pitch errors at all. The tonewheel for A 440 has sixteen teeth, and it runs at exactly 1650 RPM. This results in a speed ratio between the 1200 RPM main shaft in the Hammond tone generator and the 1650 RPM tone wheel speed of 1.375:1 which is a true number and not irrational. Thus we may obtain this correct speed accurately with gearing. But all other pitches on a Hammond organ deviate slightly from the correct frequencies, the greatest error being approximately 2 cents....negligible, forget about it!