Vibrato
North Suburban HAMMOND ORGAN Service
Here is the simplest of Leslie diagrams. It's a view looking down at the treble horns of a typical Leslie such as a 122. In a real life Leslie, there are two upper horns that are 180° apart. But only one of them works. The other is a dummy and exists only for mechanical balance so that when the horns are rotating at between 360 to 400 RPM, there will not be any wobbling, which would be severe at that speed if only a single horn was present.
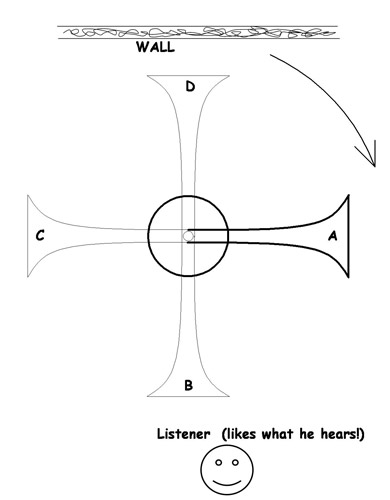
Figure 5. Here above we show one Leslie horn in four positions. We'll assume at the moment that it is in position A as indicated by the heavier lines and that it rotates in the direction indicated. A listener stands in front of the Leslie cabinet, and there's a wall behind it. As the horn rotates from position A to position B, the listener hears a slightly increased frequency of the signal, because the Doppler effect comes into play as the sound source (open end of horn) moves toward the listener. At the same time, however, the horn is moving away from the wall behind the speaker, and the frequency of the sound at the wall is slightly lower because the horn is rotating AWAY from the wall. Therefore, a sound wave reflected from the wall will have a lower frequency than that of the sound wave from the horn. The listener, however, hears both sounds, the direct signal from the horn with a slightly increased pitch, and the reflected signal from the wall with a slightly reduced pitch.
As the horn continues to rotate, eventually it will pass through position B. For the instant that the horn is in B, the listener will hear the sound at the same pitch as the original signal fed into the horn, and likewise the sound reflected from the wall will equal that of the original signal. At that brief instant, the distance between the horn and the listener and the wall is not changing. However, as soon as the horn continues even the tiniest fraction of a degree past position B and heads toward position C, the listener once again hears a signal whose pitch is slightly different from that of the original. In this case, the sound source is moving AWAY from the listener, so he hears a sound whose frequency is slightly lowered.
However, the horn as it approaches position C is also moving closer to the rear wall, so the sound wave at the wall and reflected from it has a slightly higher than normal pitch. When the horn passes through position D, for the briefest of instants the listener will hear the original pitch unaltered from both the horn itself and also from the reflected wave from the rear wall. Keep in mind however that this is an extreme simplification of what actually happens.
Referring again to the diagram, the listener in the picture will hear the greatest pitch deviation when the horn rotates through positions A and C, with a gradually decreasing pitch deviation as the horn approaches positions B and D. (This of course assumes no other sound reflecting surfaces. In actuality, the result is much more involved.)
If we're conducting these observations in a typical room which is generally the case, then there will be four walls and at the very minimum, four reflected sound waves, all of which are constantly changing in pitch as the horn rotates closer to and then away from a given wall. Add to this all of the sound waves that are reflected from various objects in the room, and even from the inside walls of the Leslie speaker cabinet itself, and you will quickly see that the resulting vibrato consists of an infinite array of different vibratos, some increasing in instantaneous frequency and others decreasing, or possibly undergoing brief intervals of no frequency shift at all. Furthermore, if the listener moves even slightly, the pattern of pitch changes that he hears will be at least a little different.
So, not only do we get an infinite array of subtle pitch changes, we also get changes in loudness, for when the horn is approaching the listener, the sound he hears will become a little louder than the sound he hears when the horn rotates away from the listener.
You can confirm all of the above yourself if you have access to a Leslie speaker. Put a small weight on one of the playing keys of the instrument with the Leslie, and then walk around the room and listen. All kinds of subtle and in some cases not so subtle variations in the resulting vibrato/tremolo mix will be apparent. Therefore we can say that no two people ever hear the same Leslie speaker exactly the same way. We can also state that the Leslie effect is essentially infinitely complex. The Leslie effect will be different, at least slightly, in every different location where the speaker is, and because of the continuous motion of the sound, the resulting signal is a stereo signal of (effectively) an infinite number of channels.
It's doubtful that even the most powerful computers in the world, all working together, could accurately calculate all of the different frequency and volume changes that occur during one complete revolution of the rotating horn in a single 122 Leslie Speaker in an average rectangular room.
Now how much of a pitch change is possible with a typical Leslie Speaker? In practice of course, the Leslie does not alter the pitch of the audio signal which it receives from the electronic organ to which it is connected. However, once the sound leaves the open end of the rotating horn, the doppler effect comes into play and accounts for most of these complex frequency shifts in the sound wave.
Anyhow, the typical top horn in a Leslie is about 10 inches long. As it rotates, it moves around in a 20 inch diameter circle. If the rotating speed of the horn is 360 RPM, then the circumferential speed of the open end of the horn is 31.42 feet per second. This is roughly 21 miles per hour. This results in a frequency shift of roughly 2.9 percent or nearly half a semitone. In other words, the maximum apparent pitch deviation that could occur from a Leslie speaker because of the doppler effect is about a quarter of a tone; that is, about half the distance in pitch from, say, C to C#.
Because the Leslie speaker produces a virtually infinite array of vibratos, all deviating in pitch at different times, a listener is not really so much aware of any particular pitch change, but of a very interesting and extremely complex variation that occurs at the typical vibrato rate of roughly 360 to 400 times a minute.
The Leslie speaker was originally developed as a means to add a much more interesting effect than the simple tremolo of early Hammond organs. After Don Leslie perfected his speaker systems, the "Leslie" sound became extremely popular because of the vast improvement it made to so many different electronic organs.
We might now consider once again the Hammond scanner vibrato and compare it to the action of a Leslie speaker, because in some ways these two completely different animation systems have some unusual parallels. Animation, by the way, seems to be a Hammond term and they use it to represent any effect such as vibrato or tremolo or even a slow scan celeste, in other words, any effect that alters the tone in some manner such as the above.
Previous Page Page 5. Next page